Dr. Glen Brown
Global Accountancy Institute, Inc. & Global Financial Engineering, Inc.

Abstract

This paper introduces the Global Adaptive Statistical Break-Even Trigger (GASBET) Model—a dynamic mechanism for setting break-even points in our proprietary Global Algorithmic Trading Software (GATS) Framework. By integrating statistical measures such as the mean and standard deviation of our Dynamic Adaptive ATR Trailing Stop (DAATS) values, the GASBET Model tailors the exit thresholds to each asset’s unique volatility profile. This adaptive approach minimizes premature stop-outs, secures unrealized profits, and enhances overall risk-adjusted returns, bringing us one step closer to an “ATM-like” trading system.

1. Introduction

In modern financial markets, efficient risk management is paramount to capture profitable moves while minimizing losses. Traditional fixed break-even triggers may fail to account for varying volatility across assets, leading to either premature exits or delayed profit protection. The GASBET Model addresses these challenges by leveraging statistical insights to dynamically set break-even thresholds based on the properties of our DAATS values.

3. The GASBET Model

3.1 Concept and Rationale

The Global Adaptive Statistical Break-Even Trigger (GASBET) Model is designed to set adaptive break-even thresholds based on the statistical distribution of our DAATS values. Rather than using a fixed percentage trigger (such as 35% of DAATS), GASBET leverages the mean and standard deviation of DAATS to adjust the break-even point dynamically.

This approach ensures that the break-even trigger:

• Adapts to Volatility: Reflects the relative volatility of each asset.
• Avoids Premature Exits: Ensures that normal market fluctuations do not trigger a premature exit.
• Maintains Consistency: Provides a unified framework across all instruments in our portfolio.

3.2 Statistical Foundation

For a given set of DAATS values, we calculate:

• Mean (μ): The average DAATS value across the portfolio.
• Standard Deviation (σ): Measures the dispersion of DAATS values around the mean.

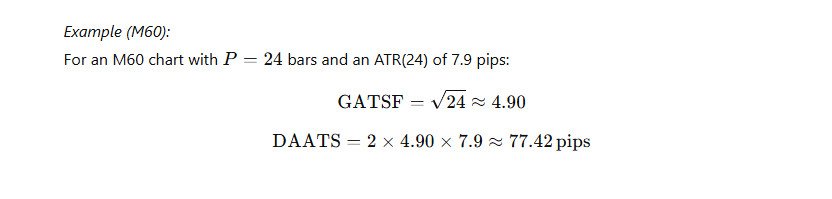
For example, assume that for a given period on the M5 timeframe for a set of currency pairs, we have:

• μ≈3232.86 points
• σ≈1160 points

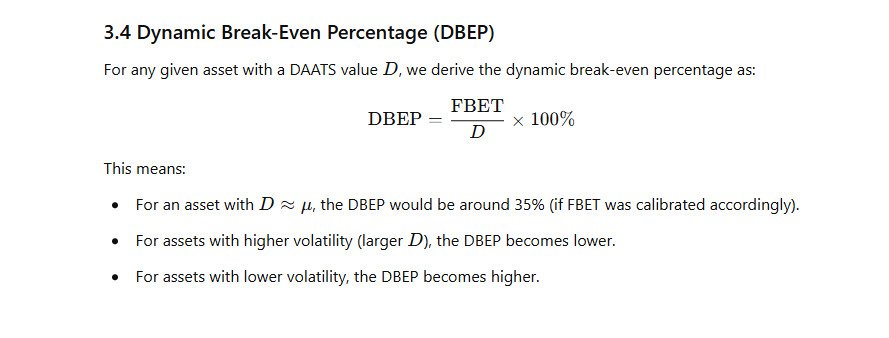
We propose a fixed break-even threshold expressed in points, determined as:

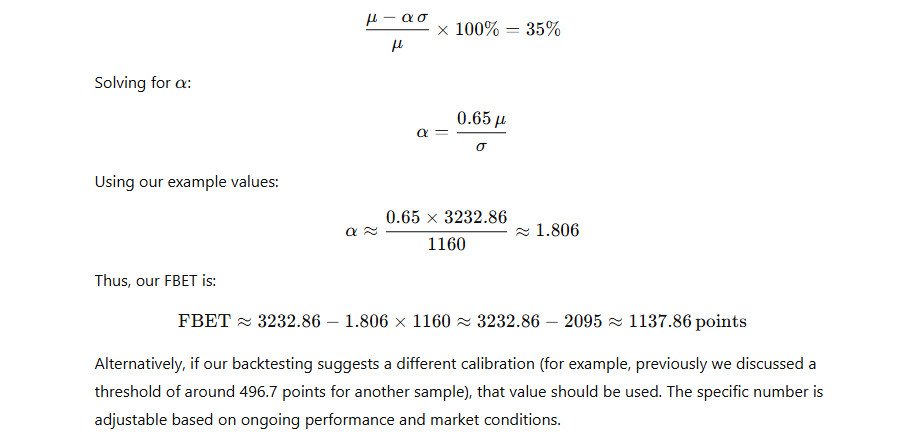
Fixed Break-Even Threshold (FBET)}=μ−ασ

Here, α is a calibration constant determined through historical backtesting to align the trigger with our target (e.g., 35% break-even for assets trading at the mean DAATS).

For instance, if we calibrate α so that an asset trading at the mean DAATS triggers break-even at 35%, we solve:

4. Strategic Applications

Trade Management

• Securing Profits:
  Once the unrealized profit reaches the DBEP (or the fixed FBET in points), the system moves the stop-loss to break-even, thereby locking in secured unrealized profits without prematurely exiting the trade.
• Adaptive Exits:
  The GASBET Model allows the exit strategy to adapt based on real-time volatility. This prevents the break-even trigger from being set too aggressively in low-volatility environments or too passively in high-volatility conditions.

Position Sizing and Risk Control

• Tailored Risk Allocation:
  By using the DBEP, we can adjust position sizes according to the volatility profile of each asset, ensuring that risk per trade remains consistent across the portfolio.
• Portfolio-Level Calibration:
  Weekly recalculation of μ and σ for DAATS values ensures that our break-even triggers remain in line with current market conditions, allowing for ongoing optimization of our risk management strategy.

5. Conclusion

The Global Adaptive Statistical Break-Even Trigger (GASBET) Model represents a significant enhancement to our GATS Framework by integrating robust statistical measures into our exit strategy. By leveraging the mean and standard deviation of DAATS values, GASBET adapts the break-even trigger to each asset’s volatility, ensuring that our stop-loss levels are neither too tight nor too loose. This adaptive approach secures unrealized profits while allowing trades the potential to reach their full target, ultimately enhancing our risk-adjusted returns and moving us closer to building an “ATM-like” trading system.

About the Author

Dr. Glen Brown is a pioneer in financial engineering and algorithmic trading. With decades of experience bridging academic theory with practical applications, Dr. Brown is the visionary founder of Global Accountancy Institute, Inc. and Global Financial Engineering, Inc. His innovative GATS Framework has set new industry standards in adaptive risk management and multi-timeframe analysis, driving consistent, superior trading performance.

General Disclaimer

The information presented in this paper is for educational and informational purposes only and should not be construed as investment advice. Trading in financial markets involves risk, and past performance is not indicative of future results. Readers are encouraged to conduct their own research and consult with a qualified financial advisor before making any investment decisions.

Global Accountancy Institute, Inc. and Global Financial Engineering, Inc. operate as closed proprietary firms. We do not offer any products or services to the general public, nor do we accept clients or external funds. All methodologies, including the GATS Framework, are exclusively developed and utilized internally as part of our proprietary trading systems.

Neither Dr. Glen Brown nor his affiliated institutions accept any responsibility for any loss or damage incurred as a result of the use or application of the information provided.